When diving into the realm of calculus and advanced mathematics, the concept of vertical asymptotes emerges as a pivotal topic that bridges our understanding of function behavior near points where the function does not behave “nicely” in a traditional sense. This article aims to demystify the process of identifying vertical asymptotes, providing a deep dive into their theoretical foundation, practical steps for finding them, and common pitfalls to avoid. By the end of this guide, you will be well-equipped with the knowledge to find vertical asymptotes with confidence, enhancing your mathematical problem-solving skills.
The Theoretical Foundation of Vertical Asymptotes
Vertical asymptotes occur where a function approaches infinity or negative infinity as the input (or x-value) approaches a certain point. This behavior signals that the function is undefined at that point, often due to a division by zero in the function’s equation. Understanding vertical asymptotes requires a grasp of limits, a fundamental concept in calculus that explores the behavior of functions as they approach specific points.
In simpler terms, a vertical asymptote represents a line (x = a) where the function’s value grows without bound as it nears ‘a’ from either side. It’s essential to note that while the function itself never touches or crosses this line, its values get infinitely close, showcasing the concept of limit in action.
Practical Steps to Find Vertical Asymptotes
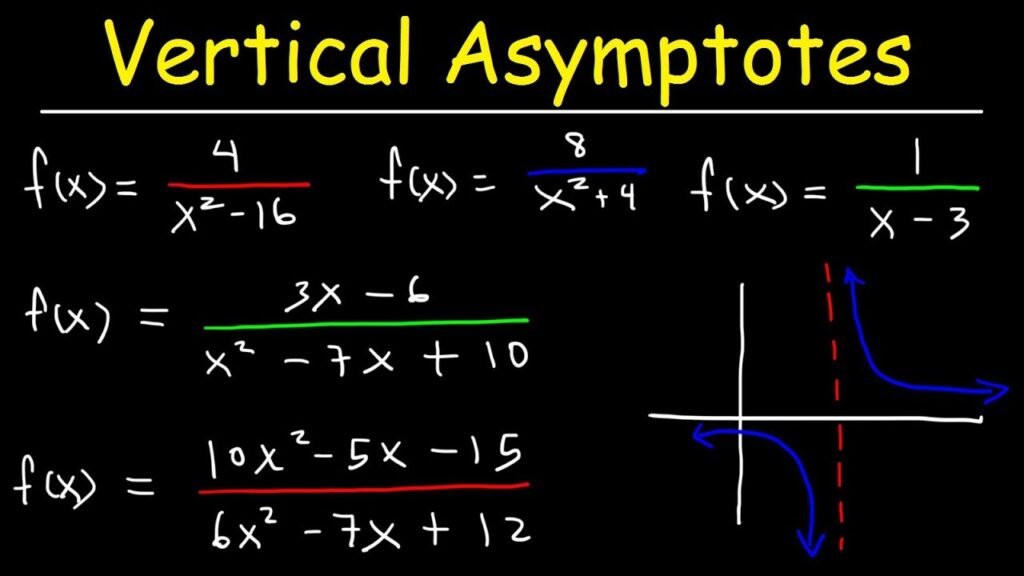
Identify the Denominator: Start by examining the function and identify any expressions in the denominator. The roots of these expressions are your potential candidates for vertical asymptotes since division by zero is undefined.
Solve for Zero: Solve the denominator’s equation for zero. The solutions to this equation are the x-values where the function could potentially be undefined, leading to vertical asymptotes.
Check the Limits: For each candidate x-value, evaluate the limit of the function as it approaches the value from both the left and the right. If the limit approaches infinity or negative infinity on at least one side, then a vertical asymptote exists at that x-value.
Consider Exceptions: It’s crucial to consider cases where a function may be undefined at a point, but a vertical asymptote does not exist. For instance, if the numerator and denominator both approach zero, further investigation is required to determine the behavior, possibly leading to a hole rather than an asymptote.
Common Misconceptions and Tips
- Not All Undefined Points Are Asymptotes: Just because a function is undefined at a point does not automatically mean there is a vertical asymptote. It’s essential to examine the limits to confirm their behavior.
- Continuous Functions: Remember, functions that are continuous over their entire domain do not have vertical asymptotes. Vertical asymptotes typically occur in rational functions where the denominator can be zero.
- Horizontal vs. Vertical Asymptotes: Do not confuse vertical asymptotes with horizontal ones. Horizontal asymptotes are concerned with the behavior of a function as x approaches infinity, not with points of undefined value in the function.
Real-World Applications and Examples
Understanding how to find vertical asymptotes is not just an academic exercise; it has practical applications in various fields such as physics, engineering, and economics. For example, in engineering, the concept of vertical asymptotes can help in understanding the behavior of stress-strain curves in materials at breaking points. In economics, they can model scenarios of rapid growth or decline, resembling “boom or bust” phenomena.
Consider the function
�(�)=1�−2
f(x)=
x−2
1
. To find the vertical asymptote, we set the denominator equal to zero:
�−2=0
x−2=0, solving for
�
x gives us
�=2
x=2. Checking the limit of
�(�)
f(x) as
�
x approaches 2, we find that the function approaches infinity, confirming a vertical asymptote at
�=2
x=2.
Conclusion
Finding vertical asymptotes is a critical skill in the mathematician’s toolbox, offering insights into the intricate behaviors of functions near points of discontinuity. By following the steps outlined in this guide and keeping an eye out for common pitfalls, you can proficiently identify and analyze vertical asymptotes. This knowledge not only enriches your mathematical understanding but also prepares you to apply these concepts in practical, real-world scenarios. Remember, the journey to mastering mathematics is filled with exploring these fascinating behaviors, each concept building upon the last to form a comprehensive understanding of the mathematical landscape.